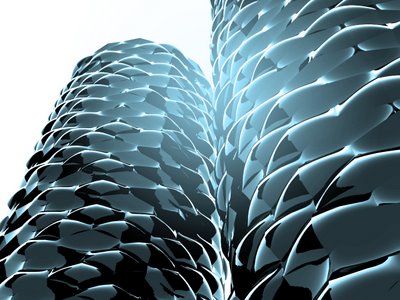
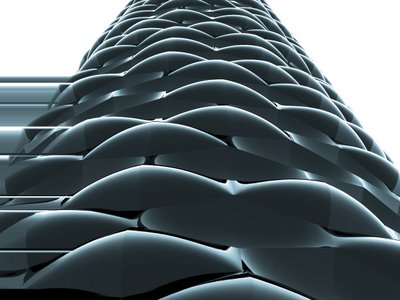
080110_Boolean_Series001

"SIDE TRACK" : CONSTRUCTIVE SOLID GEOMETRY (CSG) (ie wikipedia.org)
Constructive solid geometry (CSG) is a technique used in solid modeling. CSG is often, but not always, a procedural modeling technique used in 3D computer graphics and CAD. Constructive solid geometry allows a modeler to create a complex surface or object by using Boolean operators to combine objects. Often CSG presents a model or surface that appears visually complex, but is actually little more than cleverly combined or decombined objects. (In some cases, constructive solid geometry is performed on polygonal meshes, and may or may not be procedural and/or parametric.)

The simplest solid objects used for the representation are called primitives. Typically they are the objects of simple shape: cuboids, cylinders, prisms, pyramids, spheres, cones. The set of allowable primitives is limited by each software package. Some software packages allow CSG on curved objects while other packages do not.


It is said that an object is constructed from primitives by means of allowable operations, which are typically Boolean operations on sets: union, intersection and difference.

A primitive can typically be described by a procedure which accepts some number of parameters; for example, a sphere may be described by the coordinates of its center point, along with a radius value. These primitives can be combined into compound objects using operations like these:
- boolean union: the merger of two objects into one.
- boolean difference: the subtraction of one object from another.
- boolean intersection: the portion common to both objects

Combining these elementary operations it is possible to build up objects with high complexity starting from simple ones.


EXTEND:
in the case of spheres as primitives - if all have the same exact radius, the complex composite object -resultant from a set of boolean operations- can be describe out of one spherical mould from which all the different parts are trimmed: the challenge here will be to describe and catalogue all the parts not as geometry but rather as 3d trim paths for robotic arm...

071213_Honeycomb_Cushion

Fast track SOM "tooling": developed within the same frame work than "070720_SOM_Rhinoscripting_Class" - the code has been rapidly twiked in order to generate Honeycomb "cushions"; applied onto a primitive geometry type of host - here onto a cylindre - all the cushions are the exact same, except for their orientation: randomly inward or outwoard (therefore two moulds would still be required)

REFERENCE:
Somehow - within that generic test - the bump effect displayed here calls for an obvious references to Herzog and Demeuron's Prada building (though diagrid - glass) or Munich stadium (once more diagrid - inflated ETFE cushions)


PARAMTERS:
- honeycomb vertices in a row
- honeycomb vertices in a column
- depth for the "cushion"
- target percentage for the random number of cushions oriented outward or inward

"SIDE TRACK": ETFE (ie: wikipedia.org)
ETFE (Ethylene Tetrafluoroethylene) is a fluorocarbon-based polymer (a fluoropolymer): a kind of plastic. It was designed to be a material with high corrosion resistance and strength over a wide temperature range.

An example of its use is as pneumatic panels to cover the outside of the football stadium Allianz Arena or the Beijing National Aquatics Centre - the world's largest structure made of ETFE film (laminate). The panels of the Eden Project are also made of ETFE and the Tropical Islands have a 20.000 m² window made of this translucent material.

ETFE is commonly used in the Nuclear Industry for tie or cable wraps. This is because ETFE exhibits excellent mechanical toughness and a chemical resistance that rivals Polytetrafluoroethylene (PTFE). In addition, ETFE exhibits a high-energy radiation resistance and can withstand moderately high temperatures for a long period of time.

Examples of brand names of ETFE are Tefzel by DuPont, Fluon by Asahi Glass Company and Texlon by Vector Foiltec.